🐿️ Squirrel robot

Last updated on Mar 2, 2025 Project

A squirrel robot for studying squirrel’s aerial righting ability.

Table of Contents

Key Features

🐿️ Behavior Analysis: Squirrels recover from unexpected falls using tail movements.
💻 Simulation Analysis: Body rotation is transferred to tail rotation (conservation of angular momentum).
🤖 Robot Analysis: The robot achieves aerial righting using squirrel’s control strategies.

Overview

Squirrels inhabit environments where they frequently need to control their posture in mid-air as they leap from tree to tree. A went-viral YouTube video captures a squirrel unexpectedly being launched from a platform and successfully landing on its feet by skillfully using its tail to counteract body rotation. The squirrel first moves its tail to stabilize head rotation, allowing it to visually track the landing site accurately. Next, it spins its tail to control its body’s rotation. Through computer modeling and a squirrel-inspired robot, research has revealed that a tail weighing only 3% of the total body weight can be used inertially to shift angular momentum from the body to the tail, effectively suppressing rapid body rotation. This discovery highlights the critical role of the tail in ensuring safe landings in complex arboreal environments.

Video

Observation and Behavior Analysis

A video posted on @MarkRober’s YouTube channel (see below) shows a parkour squirrel being suddenly launched from a platform. Initially, its body rotation was uncontrolled, but the squirrel skillfully moved its tail, stabilized its posture, and ultimately landed safely.

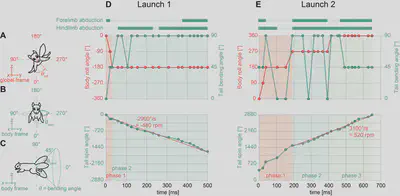
Frame-by-frame analysis of this video allowed us to closely observe the squirrel’s behavior. At first, the squirrel coordinated its body and tail movements to recover from uncontrolled rotation while stabilizing its head and visually fixing its landing site (Phase 1). Then, it continued spinning its tail to generate reaction momentum, decelerating its body rotation and eventually stopping to prepare for landing (Phase 2).

Observation Results of Squirrel's Aerial Posture Control — Observation Results of Squirrel’s Aerial Posture Control

Modeling and Simulation Studies

Squirrel Physical Model

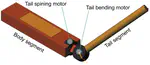
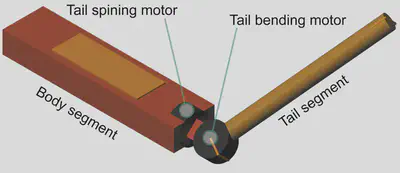
Next, we created a simulation model of a squirrel. We quantified the segmental mass and dimensions of Sciurus carolinensis (Eastern gray squirrel) specimens and used MATLAB/Simulink Simscape Multibody to build a physics-based model (see figure below). This model includes two degrees of freedom (DOF) for controlling tail flexion and spinning, both driven by motor torque. The first DOF connects to the body, while the spinning motor is mounted on the output shaft of the flexion motor.

Click here to display the parameter table

Model parameter

Parameter	Variable	Axis	Value
Body mass (kg)	$m_\mathrm{b}$	–	$0.330$
Tail mass (kg)	$m_\mathrm{t}$	–	$0.011$
Body dimensions (m)	$l_\mathrm{bl}$	length	$0.236$
	$l_\mathrm{bw}$	width	$0.056$
	$l_\mathrm{bh}$	height	$0.03$
Tail dimensions (m)	$l_\mathrm{tl}$	length	$0.215$
	$l_\mathrm{tw}$	width (diameter)	$0.020$
Body moment of inertia about body CoM (⁠kg·m^2⁠)	$I_\mathrm{br}$	Roll	$1.11 × 10^{-4}$
	$I_\mathrm{bp}$	Pitch	$1.55 × 10^{-3}$
	$I_\mathrm{by}$	Yaw	$1.62 × 10^{-3}$
Tail moment of inertia about tail CoM (⁠kg·m^2⁠)	$I_\mathrm{tl}$	Longitudinal	$4.26 × 10^{-5}$
	$I_\mathrm{ts}$	Cross-sectioned	$5.50 × 10^{-7}$

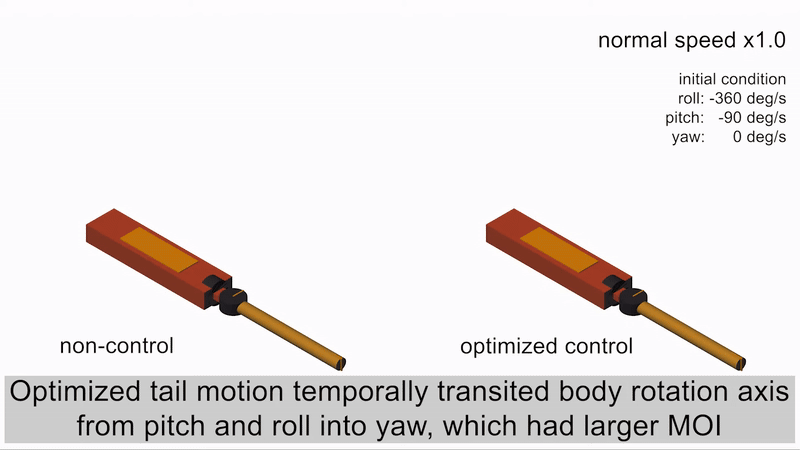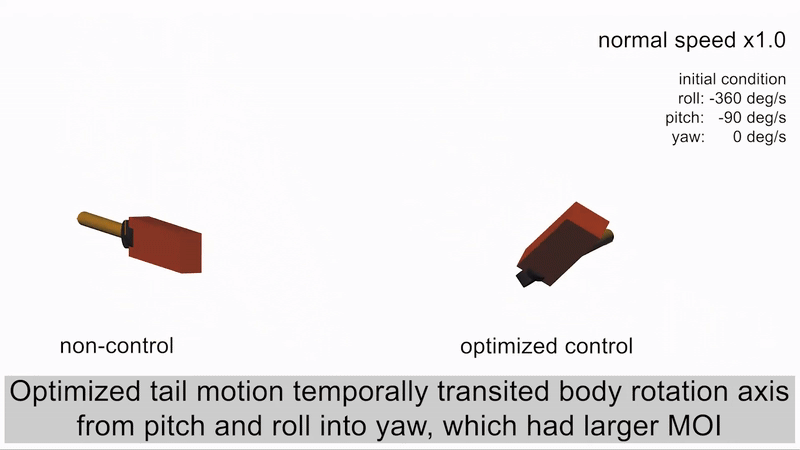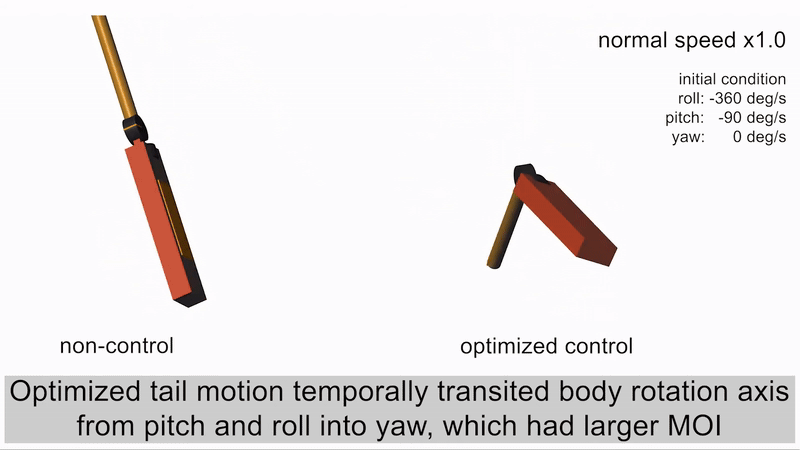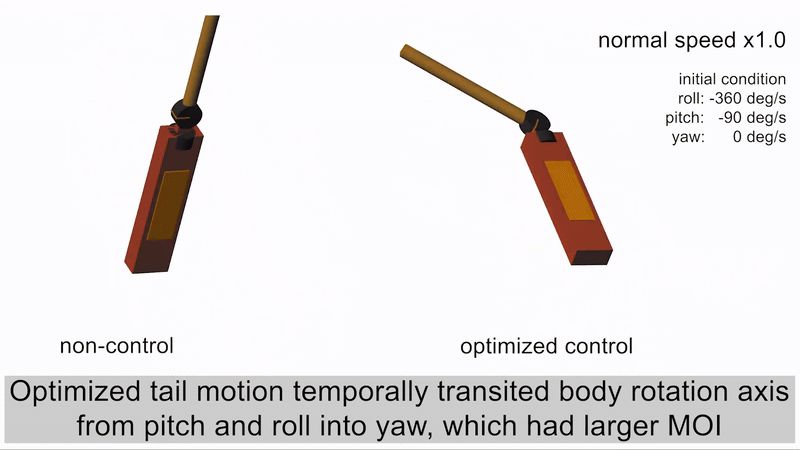
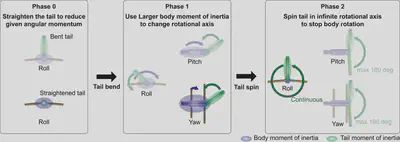
Shift of Rotation Axis from Roll & Pitch to Yaw (Phase 1)

By rapidly bending the tail from a straight position, the main rotation axis shifts from roll & pitch to yaw. Since the yaw axis has the largest moment of inertia, the rotation speed decreases according to the conservation of angular momentum, allowing the squirrel to secure more time to gaze the landing site.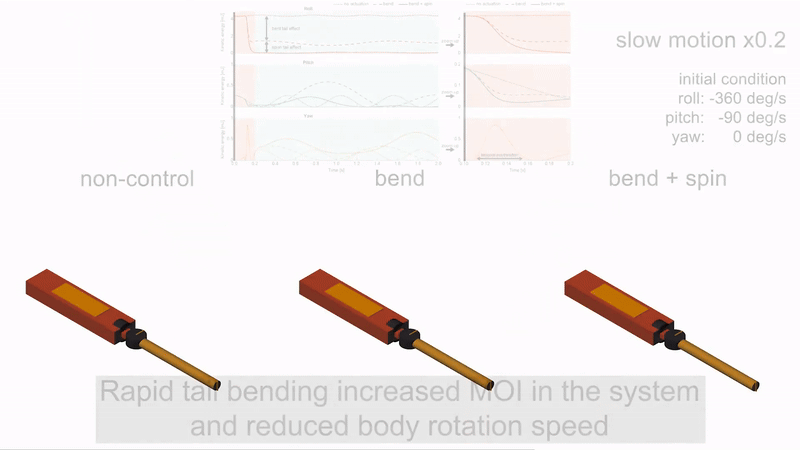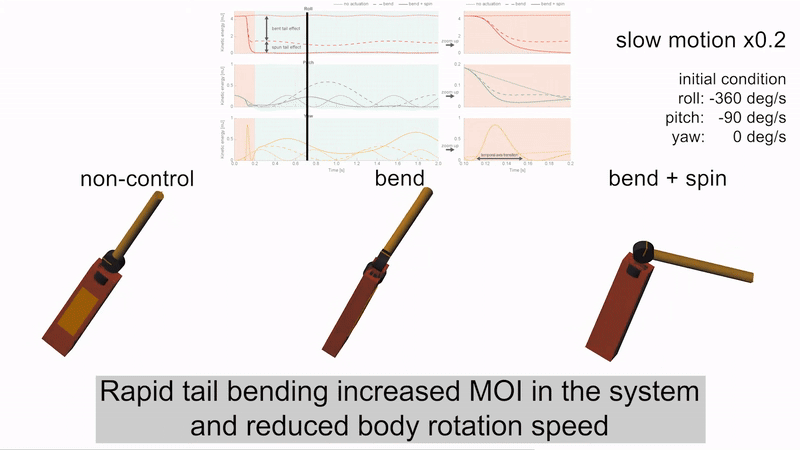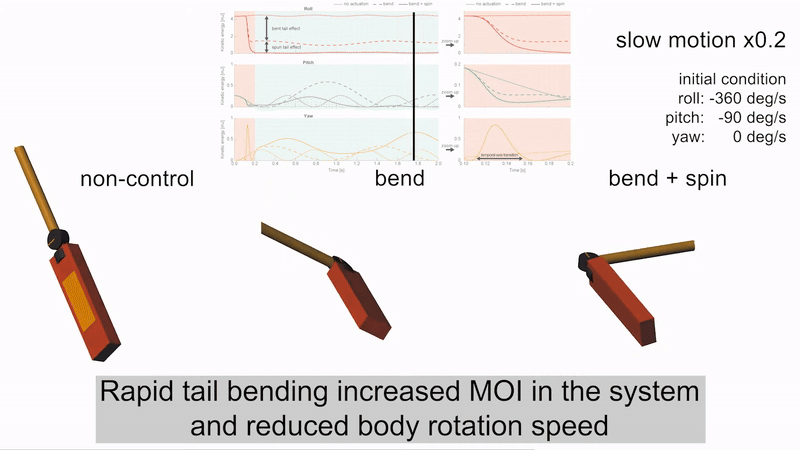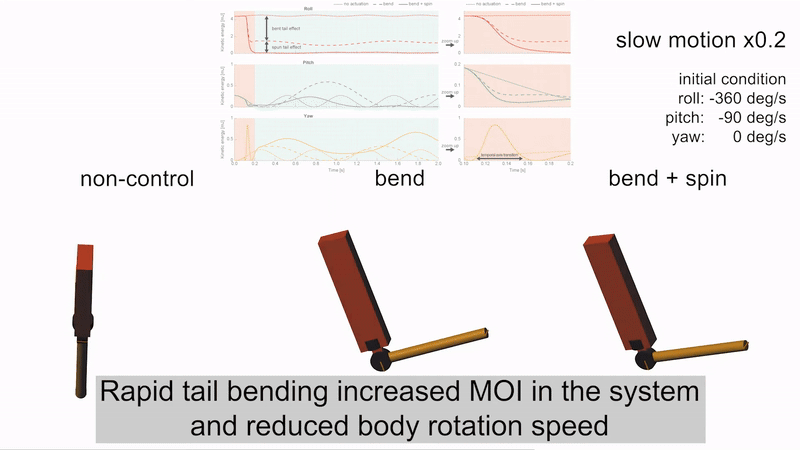

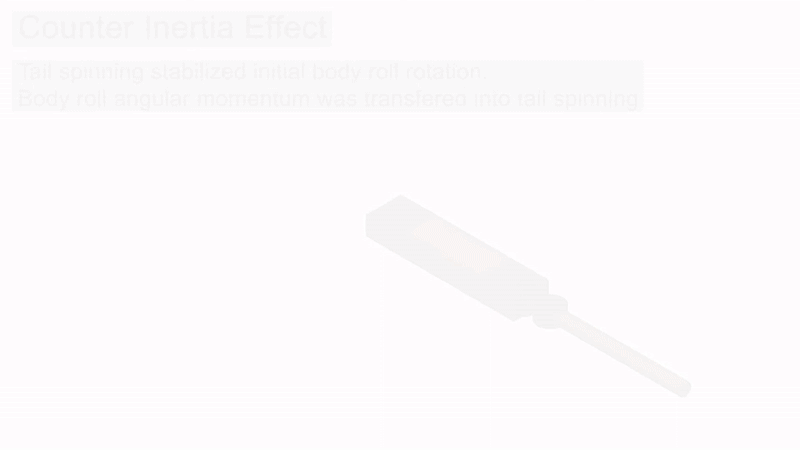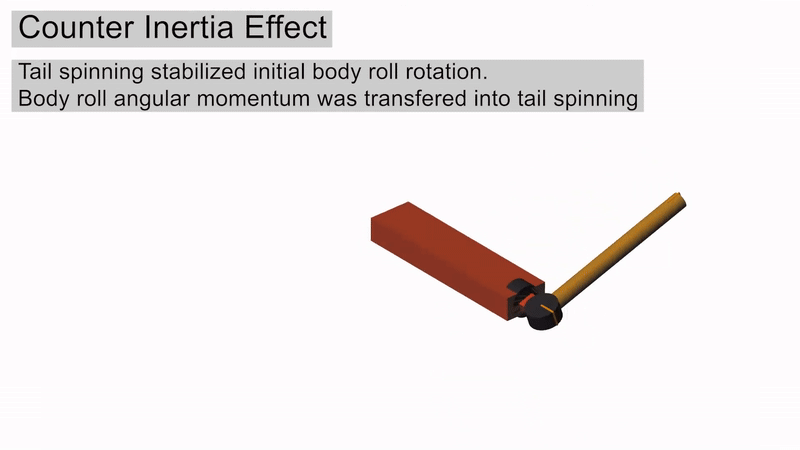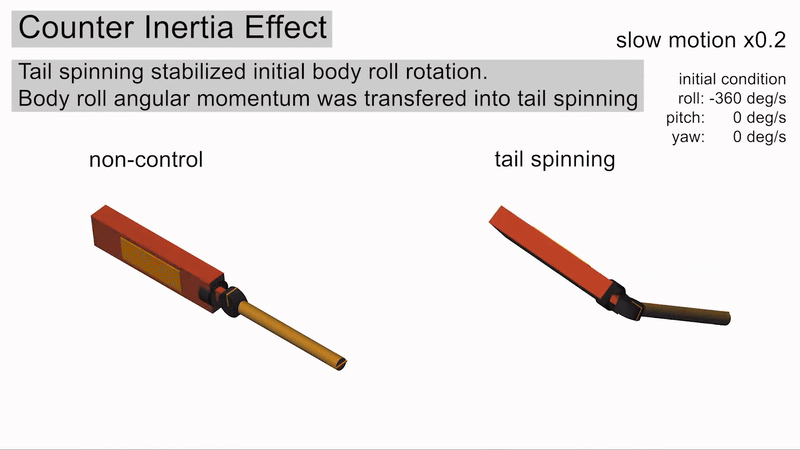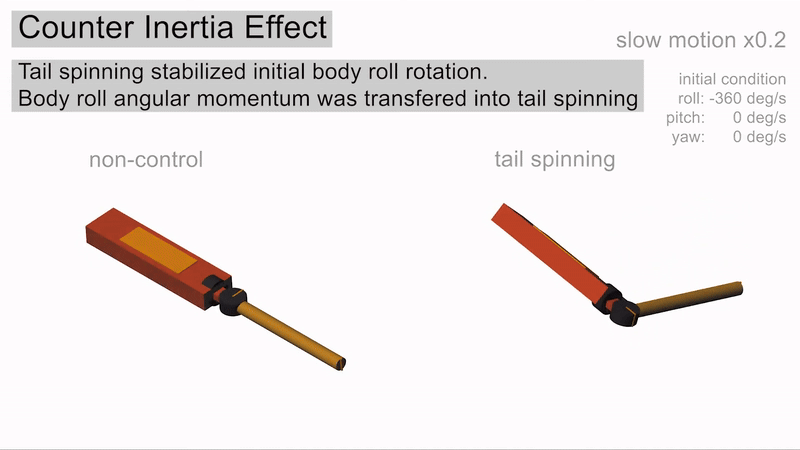
Transfer of Angular Momentum from Body to Tail (Phase 2)

By spinning the tail from a stationary state, angular momentum transitions from the body to the tail, resulting in a decrease in body rotation speed.

$$ I_\mathrm{br} \omega_\mathrm{init} + I_\mathrm{ts}\omega_\mathrm{init} = I'_\mathrm{tl}\omega_\mathrm{req} $$ $$ I'_\mathrm{tl} = I_\mathrm{tl} + m_\mathrm{t} \left( \frac{1}{2} l_\mathrm{tl} \right)^2 $$

$\omega_\mathrm{init}$: initial body (+tail) rotation speed, $\omega_\mathrm{req}$: required tail spinning speed to stop body rotation

We also found that the unique morphology of the squirrel tail contributes to improved rotational stability. Although the mass of the squirrel’s tail is only 3.3% of its body weight, the length of the tail is 91% of its body length. Thanks to this unique morphology, straightening the tail (reducing the system moment of inertia of the roll axis) when encountering an unexpected fall can reduce the required tail spinning speed to stop the body rotation by 60%.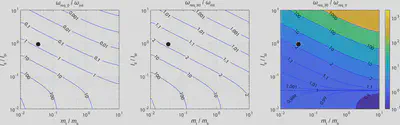

Required tail spinning speed / initial rotation speed, Left: starting with the straighten tail, Center: starting with the bent tail, Right: ratio of the straighten vs the bent tail for the required spinning speed, Black dot: squirrel's tail morphology — Required tail spinning speed / initial rotation speed, Left: starting with the straighten tail, Center: starting with the bent tail, Right: ratio of the straighten vs the bent tail for the required spinning speed, Black dot: squirrel’s tail morphology

Squirrel’s strategy

Robot & Experimental Study

Robot design

To replicate the squirrel’s aerial righting in a robot, we developed a squirrel-inspired robot with an active tail. The mass and length ratio of the body and tail were set to match real squirrel measurements (body length: 92%, tail weight: 3.4%). The robot’s body and tail are connected via a 2×1 degree-of-freedom (DOF) servo joint, allowing independent bending and spinning actuation of the tail. The bending joint is actuated by a digital servo, and the spinning joint is driven by a BLDC motor with a motor driver. This robot operates stand-alone, with a 22.2V LiPo battery and an IMU mounted on the body and a Teensy 4.1 board is used for control and data collection.

Click here to show a parameter table

Parameter	direction	value
Body mass (g)	–	415
Tail mass (g)	–	14
Body dimension (mm)	l, w, h	190, 70, 60 (excl. feet parts)
Tail dimension (mm)	l, w	175, 25 (at the counter mass ball)

Experimental result

When the robot was dropped from a height of 3.5 meters with an initial roll angular velocity, it successfully used tail movement to stop the body’s rotation. As results, angular momentum of the body was transferred to the tail, consisting with observations in real squirrels and predictions from simulations.